New approach for visualising complex mathematic topics
Since this academic year both lecturers and students can benefit from a new form in teaching and learning complex mathematic topics.
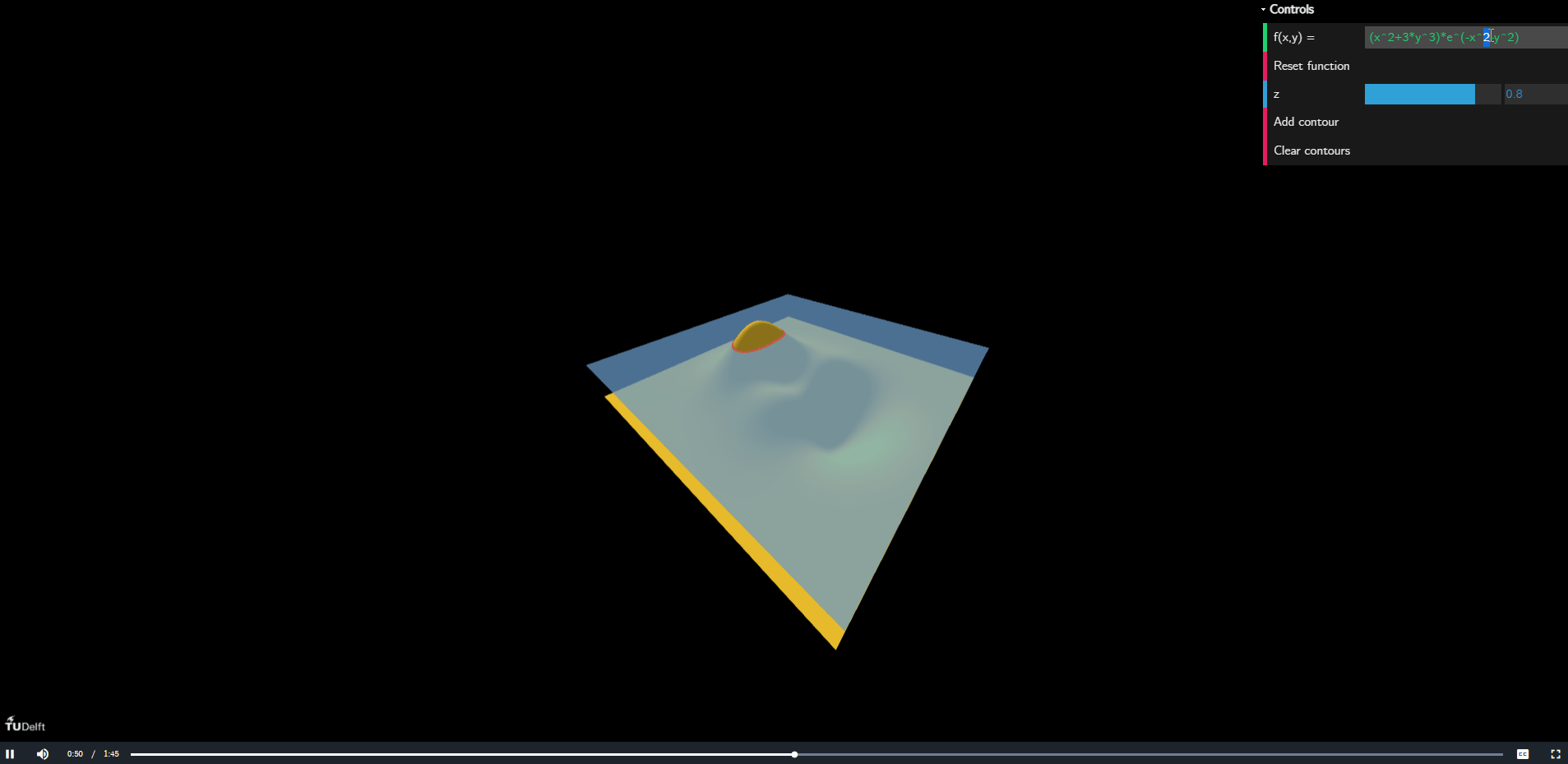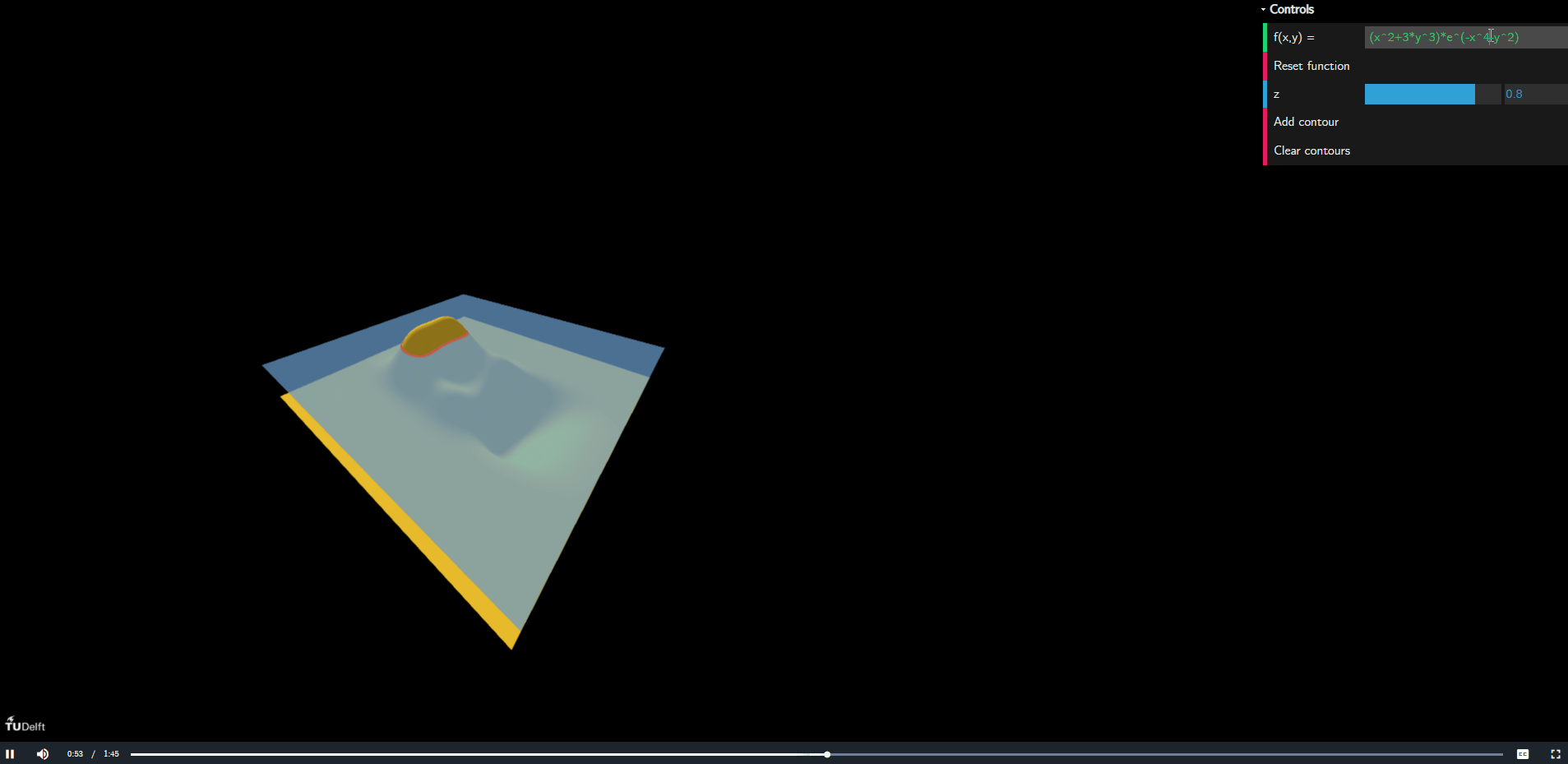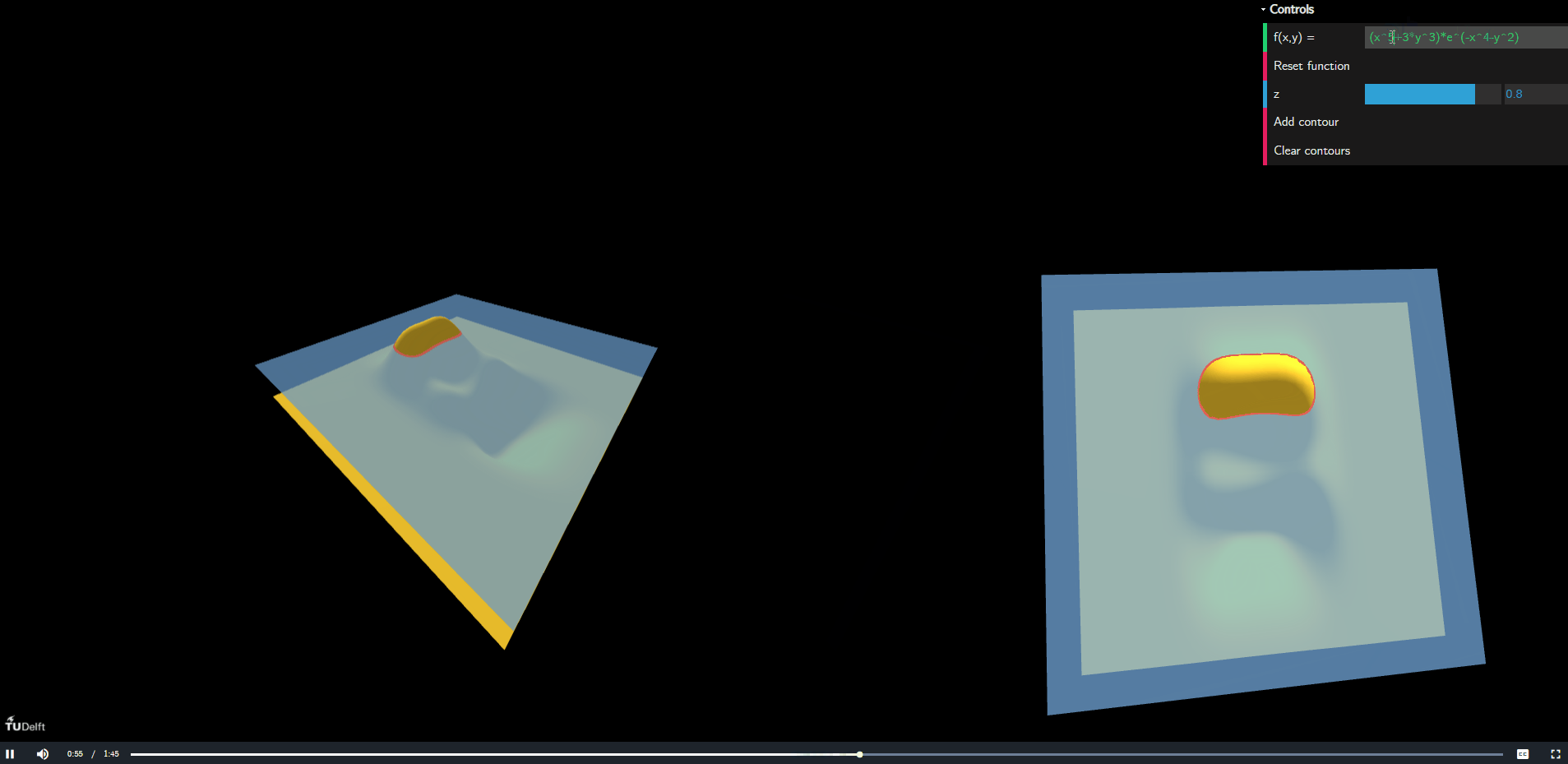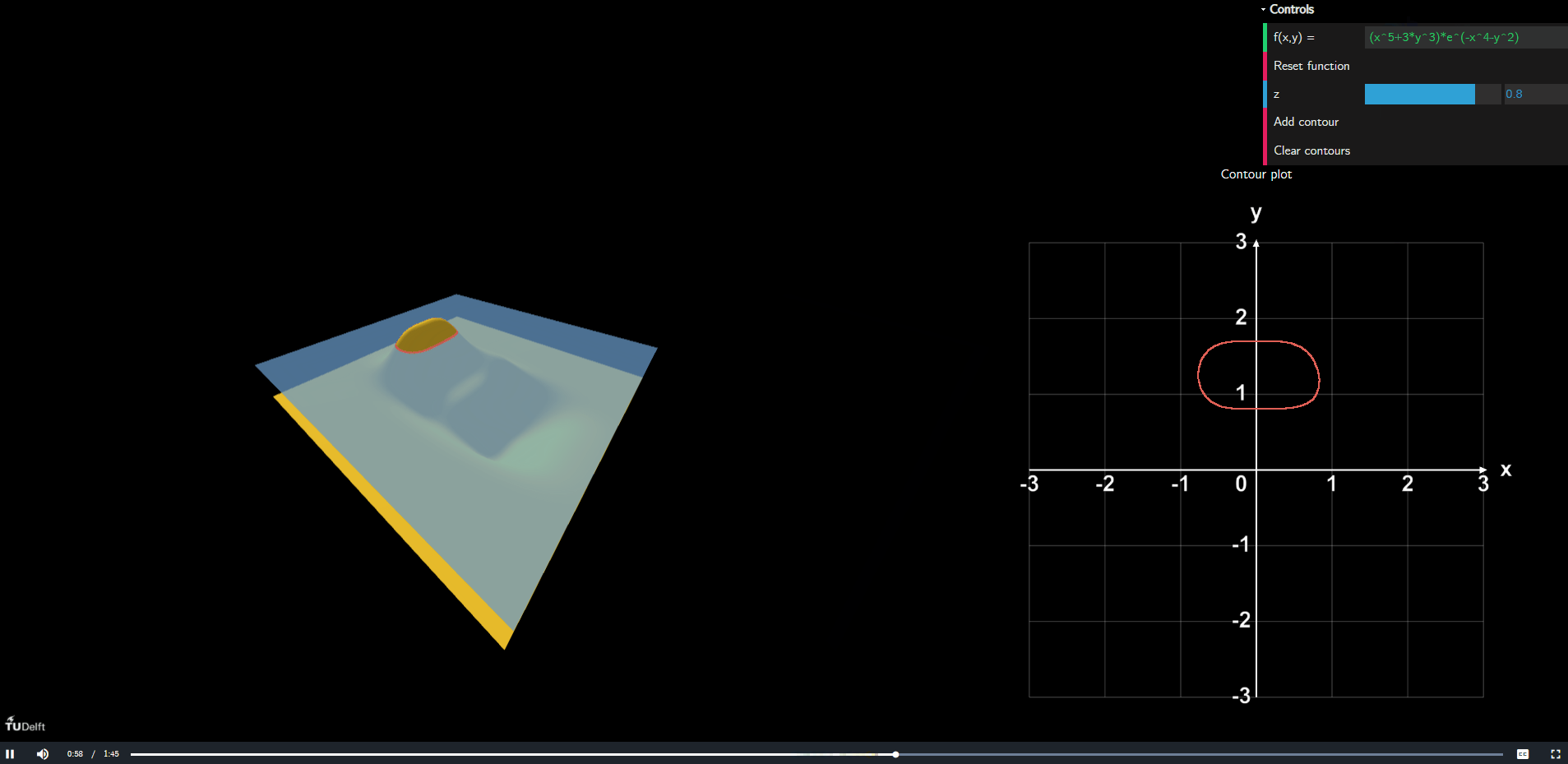
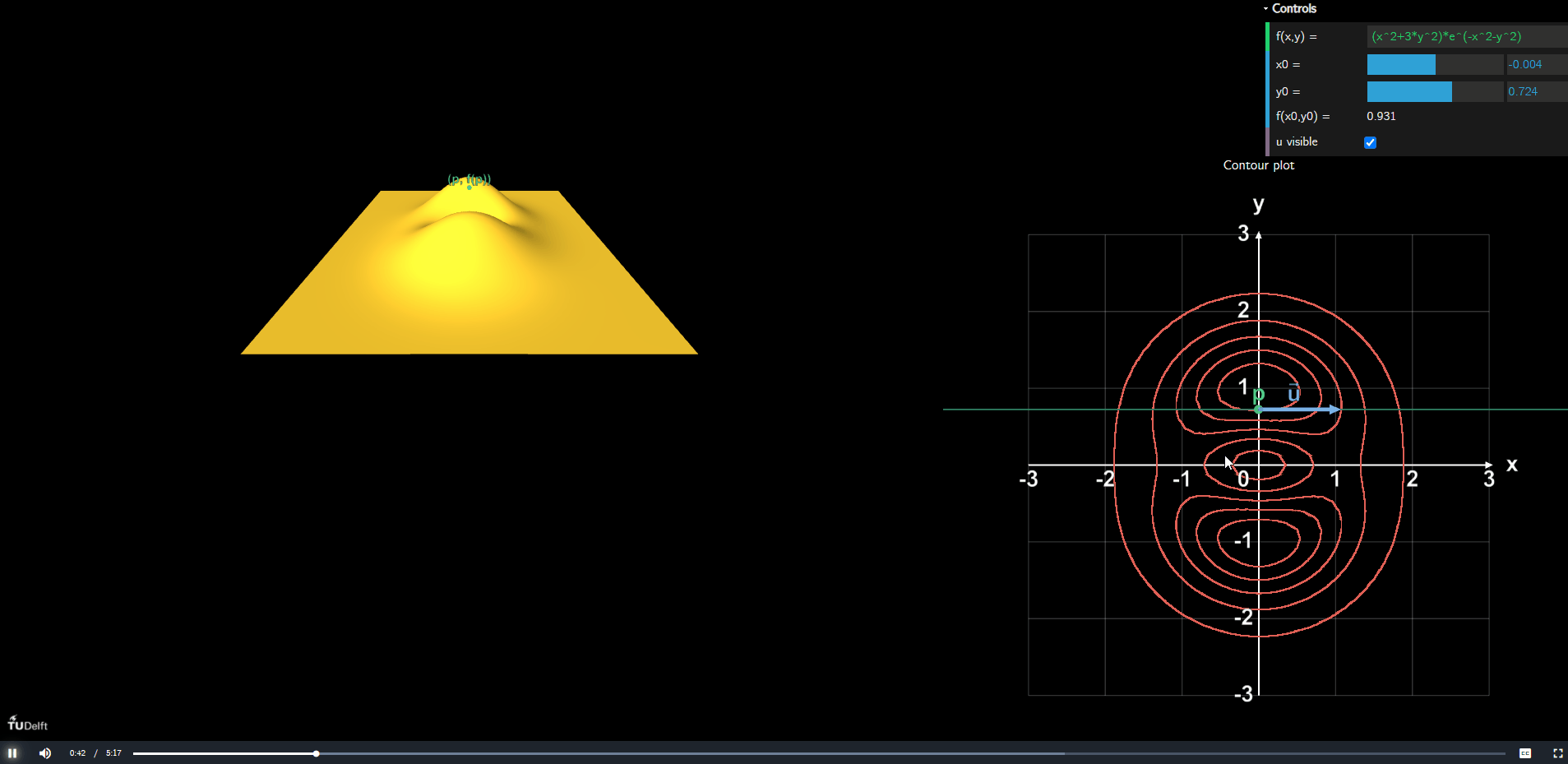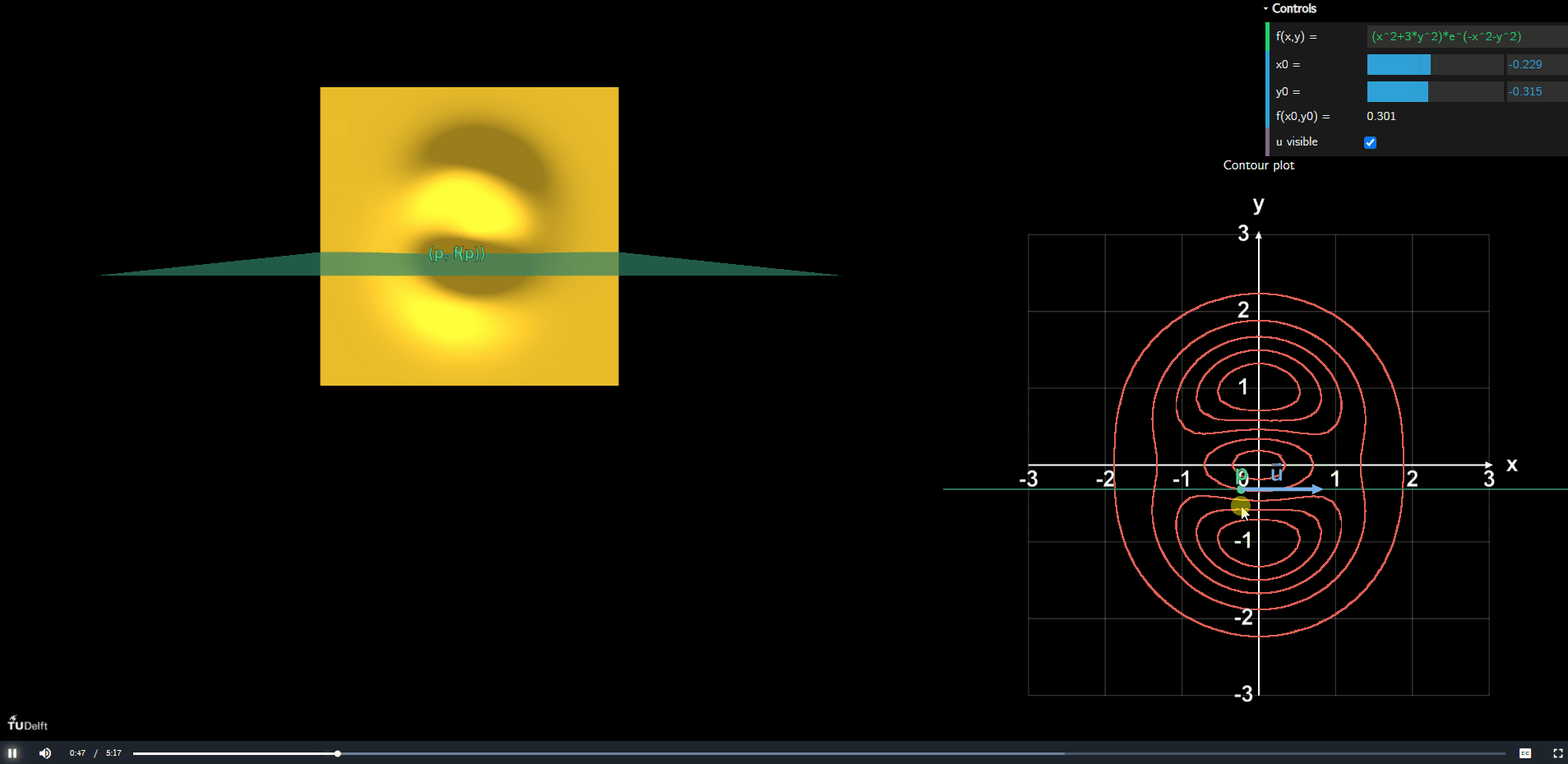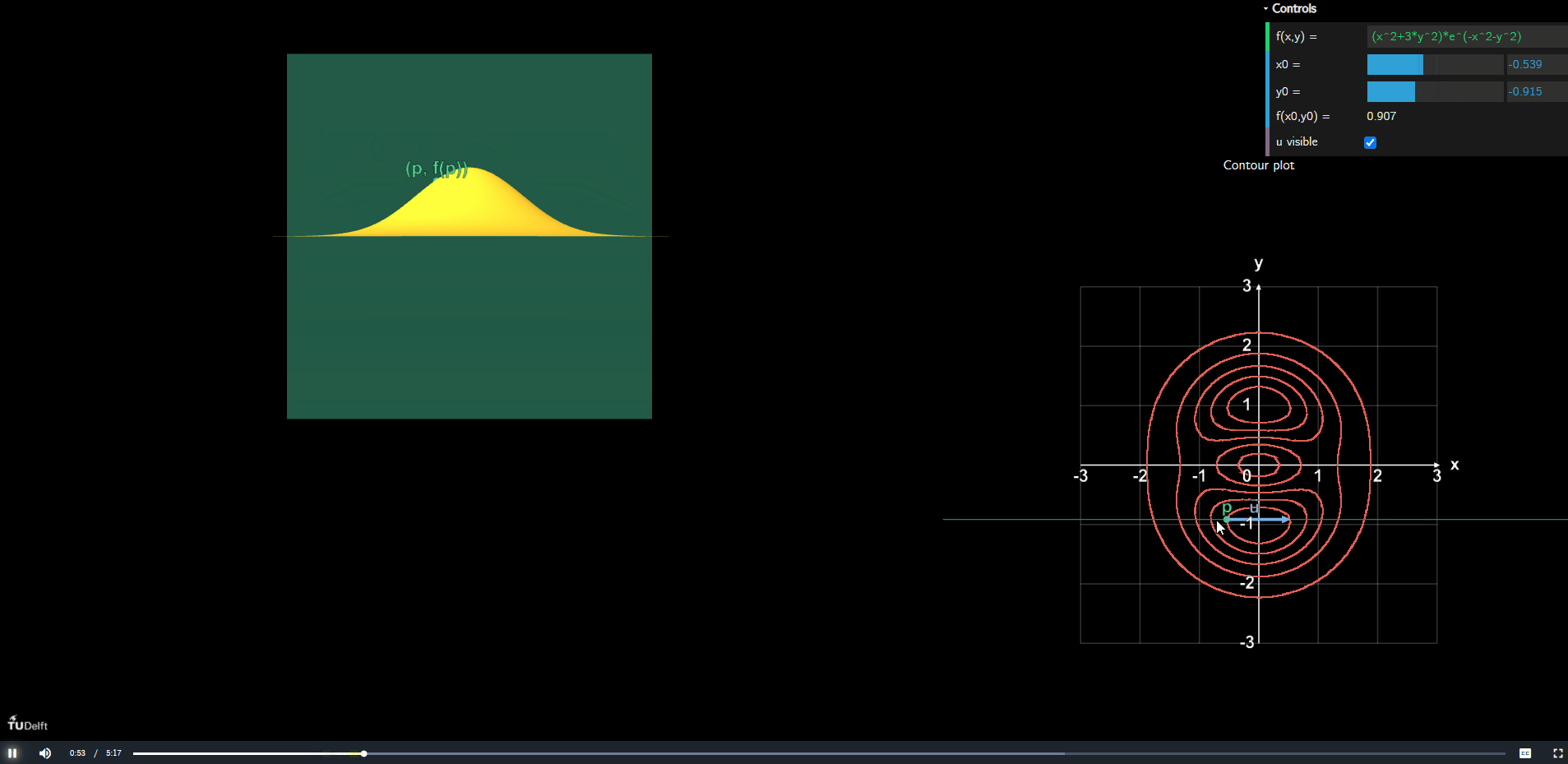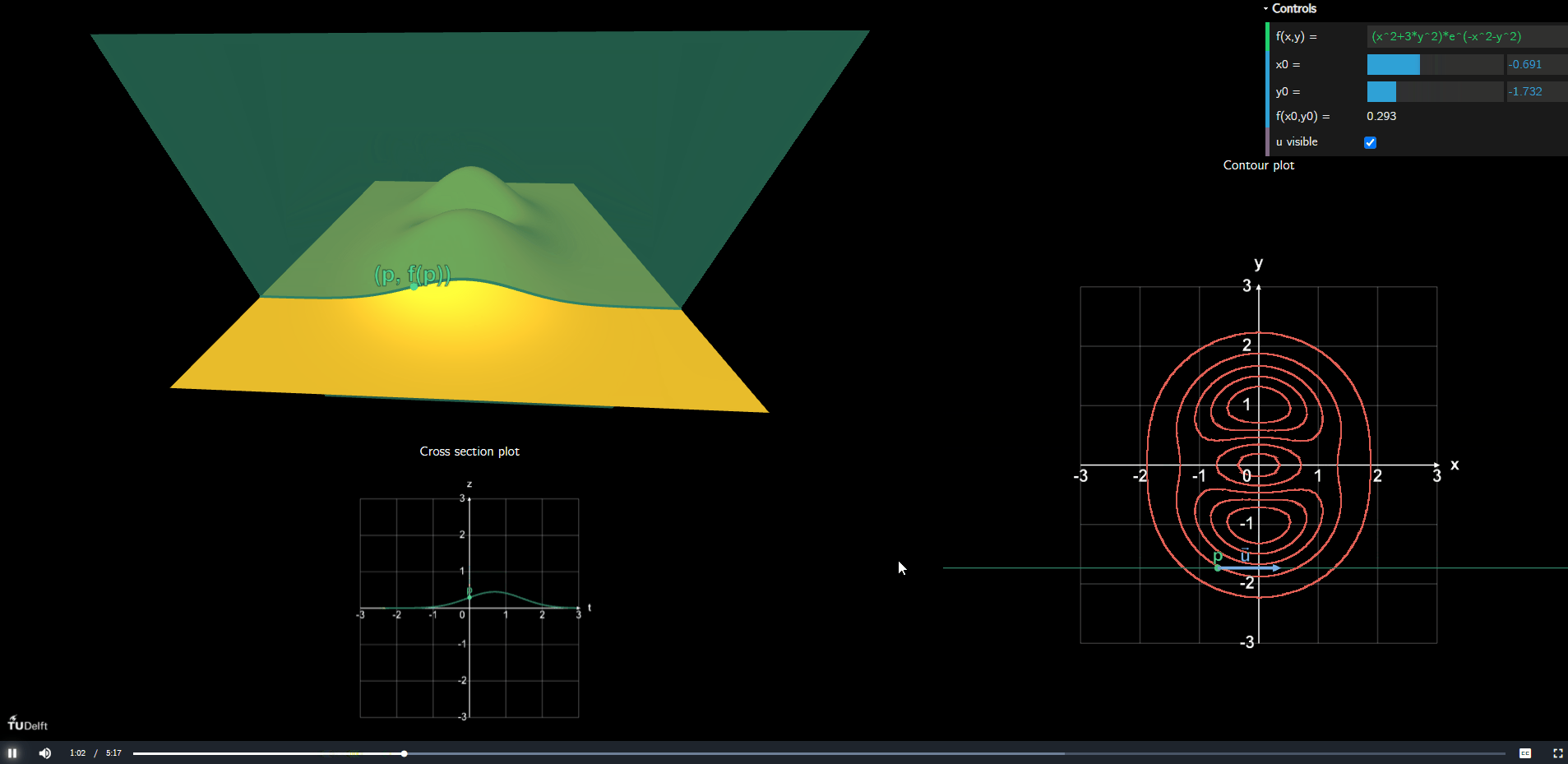
The interfaculty mathematics education team PRIME has developed a novel visualisation approach with an interactive animation. the aim of the interactive video is to increase the intuition behind the complex subject by showing a geometric interpretation. The new approach is very handy for visualising complex topics during and after class that cannot be easily sketched or drawn in a dynamic and interactive manner. Users can, for example, change the function that is plotted, drag points of interest or change the camera angle. . The animated video can be interacted with while it is paused or playing. Lecturers can use the interactive animations, usually while on 'pause', to explain and illustrate their lecture. Students can explore the interactive animation afterwards at home to recap and expand their understanding of the lecture, and use the interactive animations to do their homework exercises. Accompanying exercises have been made for students in the Grasple platform.
The approach has been used to develop a short series on directional derivatives and level curves. This is a topic taught in first-year Calculus courses. The series is now being used in most calculus courses for engineering students at TU Delft.
To experience what an interactive animation is, we recommend exploring the animations yourself. The short series can be explored via the following links:
Part 2 on Directional derivatives
Part 3 on Directional derivatives & level curves
Part 4 on Gradient & level curves
Do you want to know more about how this was made and how it is used? We did a non-technical talk about the development that you can watch online here.
Do you want to use this in your class? Feel free to do so! Please share your experiences using the series with us. We are currently working on the next series of interactive animations on Lagrange multipliers. If you have suggestions for other uses, we'd love to hear about it as well: PRIME@tudelft.nl.